Overview

The curriculum plan

You can access the latest version of the curriculum plan here, where you will find detailed

• the content of each unit
• how representations are threaded throughout the curriculum
• how concepts are developed over the curriculum

---

Sequencing

The content of the curriculum is divided into four broad strands: Number & Proportion (NP), Algebra (A), Geometry & Measures (GM), and Statistics & Probability (SP). Within each strand, content is further divided into units, with a total of 51 units spanning Years 7 to 11. Complete coverage would take a student from the start of Year 7 to full coverage of all content at Higher GCSE by the end of Year 11.

Careful, considered and deliberate thought has been given to the precedence inherent in the knowledge contained in each unit. Maths is a broadly hierarchical subject and this natural hierarchy has been exploited in the curriculum design to two ends:

1. Content in units deliberately and explicitly builds upon and uses content from preceding ones.
2. Units can be sequenced in numerous ways to fit your timetable provided you don’t break certain orders of precedence.

---

The precedence table looks a bit like this and can be found on the latest version of the curriculum plan.

---

---

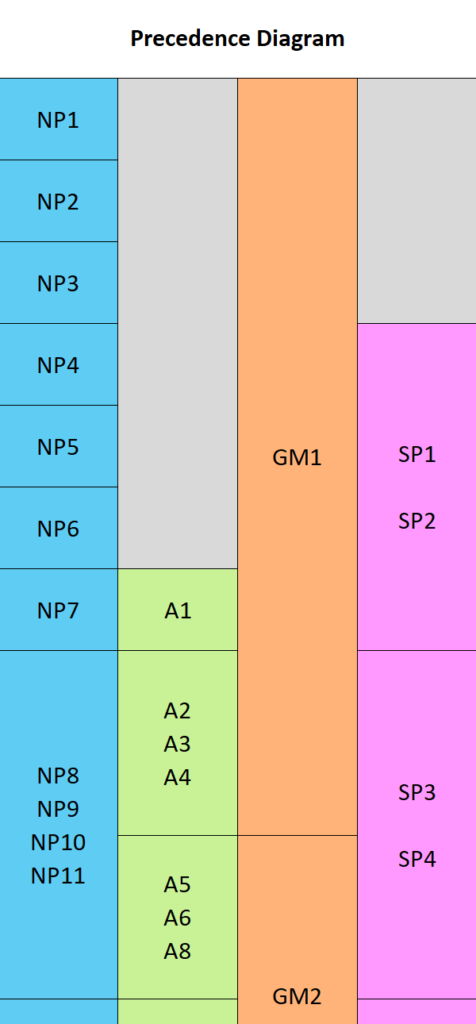
Unit NP6 (Directed Numbers) must come after NP5 (Order of Operations). A2 (Manipulating and Simplifying Expressions 1) cannot happen before NP7 (Fractions) as it will use fractions in the tasks.

GM1 (Drawing, Measuring and Constructing) can go anywhere provided you don’t start GM2 (Polygons and Angles) before A4 (Linear Equations) has been done, since the former will incorporate some element of solving equations.

There are currently schools using these resources in many ways. Some have mixed-attainment or wide-attainment classes, some have setted classes. We are clear that all students should be taught maths at a level appropriate to their current attainment and moved on at a pace that allows them the greatest success. We do advocate a one-size-fits-all approach to curriculum.

Research in mathematics education and cognitive science

Particular care and attention have been paid to what we know about learning mathematics from research as well as evidenced principles from cognitive science, such as interleaving and distributed practice. Tasks and activities are designed to subordinate previously-learned content to new, incorporating prior learning into new wherever possible in order to improve retention and recall and the ability to connect knowledge across topics.

The tasks in the booklet are sometimes designed in-house and sometimes taken from other sources. This curation of tasks only happens with strict adherence to our overriding curricular decisions: no task is included unless it contributes to the journey we have chosen for our students.

Didactics and pedagogy

We have made specific decisions about the types of representations we use, and particular methods that should be taught. This is to allow adherence to the journey we are designing: we want every experience in the classroom to contribute towards the mental schema of mathematics that we are trying to carefully and gradually help students to build. By choosing particular representations (such as the number line and algebra tiles) and making their use regular, consistent and clear, we are giving students tools with which to think about an abstract subject but, importantly, allowing them the exposure and time to assimilate these structures and become competent with them. For this reason, we believe that a whole-hearted embrace of the curriculum will be more successful than periodic toes-in-the-water, however, we present the materials here in the hope that you can make good, thoughtful use of them even if you choose not to follow the curriculum entirely.

We believe that students must learn in a distraction-free environment, and that high expectations (particularly on the responsibility of students to work hard and think hard) should be at the forefront of every maths lesson. Children are capable of achieving highly and producing excellent work; we must not lower our expectations of them. Every child deserves regular and consistent opportunities to think mathematically, to reason and to make sense of mathematical concepts. We have built this as much as possible into our resources, but there is still an imperative on the teacher to teach in a way that creates these opportunities and nurtures mathematical thought. How we teach mathematics and link knowledge to what children already know is essential and any bank of resources such as this can come to life or fall flat depending on how they are used in the classroom.

We caution against breaking the curricular resources down into a lesson-by-lesson sequence (e.g. Lesson 1, Lesson 2, Lesson 3, etc). This approach builds in artificial barriers that can reduce progress. Think of each unit as a learning sequence and take students on a journey through the material that is appropriate to them. Curriculum-led time is better than time-led curriculum.

Adapting the curriculum

We have tried to make resources that form a high-quality and near-comprehensive baseline for you. It is essential that you never just click and play. You must adapt what is given to suit your classes. Some classes will take five minutes on a task that may take others an hour. Some classes will need more practice in the basics than these materials provide. Some classes may move through the materials at a faster rate than others. You must know your students and make these judgement calls as you go.

Ultimately, it’s better not to rely on a PowerPoint to teach maths, but we have presentations here as a safety net and as professional development – helping you to get ideas for teaching the material, for the kind of rigour in language and ideas that we should all develop, and for the ways we can teach concepts and processes coherently. There is no expectation that these presentations must be used if you want to follow the curriculum, but be aware that they are there to reduce your workload, allowing you to spend your time thinking about and preparing your lessons rather than making materials.

Resources

Each unit is resourced with a sequenced booklet of tasks and a unit-long presentation that can be adapted for use in the classroom. The tasks are a combination of curation and creation that we believe fits our particular curricular journey through the mathematics of Key Stages 3 and 4. It is essential that mathematics is never taught by ‘click-and-play’ through a presentation, but we know that many teachers would benefit from a starting point rather than having to plan from scratch. To this end, we have made the booklets and presentations to reduce workload, to make suggestions for teaching, and to allow teachers time to prepare how to make it work in the classroom. There is no expectation that everything be used exactly as it is, in fact, we believe quite the opposite – that every teacher can use the same core materials but must make them come alive for their class.

Credits

View all…